Bi-Directional Polarised Light Transport

Michal Mojzík
Charles University, Prague Tomáš Skřivan
Charles University, Prague Alexander Wilkie
Charles University, Prague Jaroslav Křivánek
Charles University, Prague

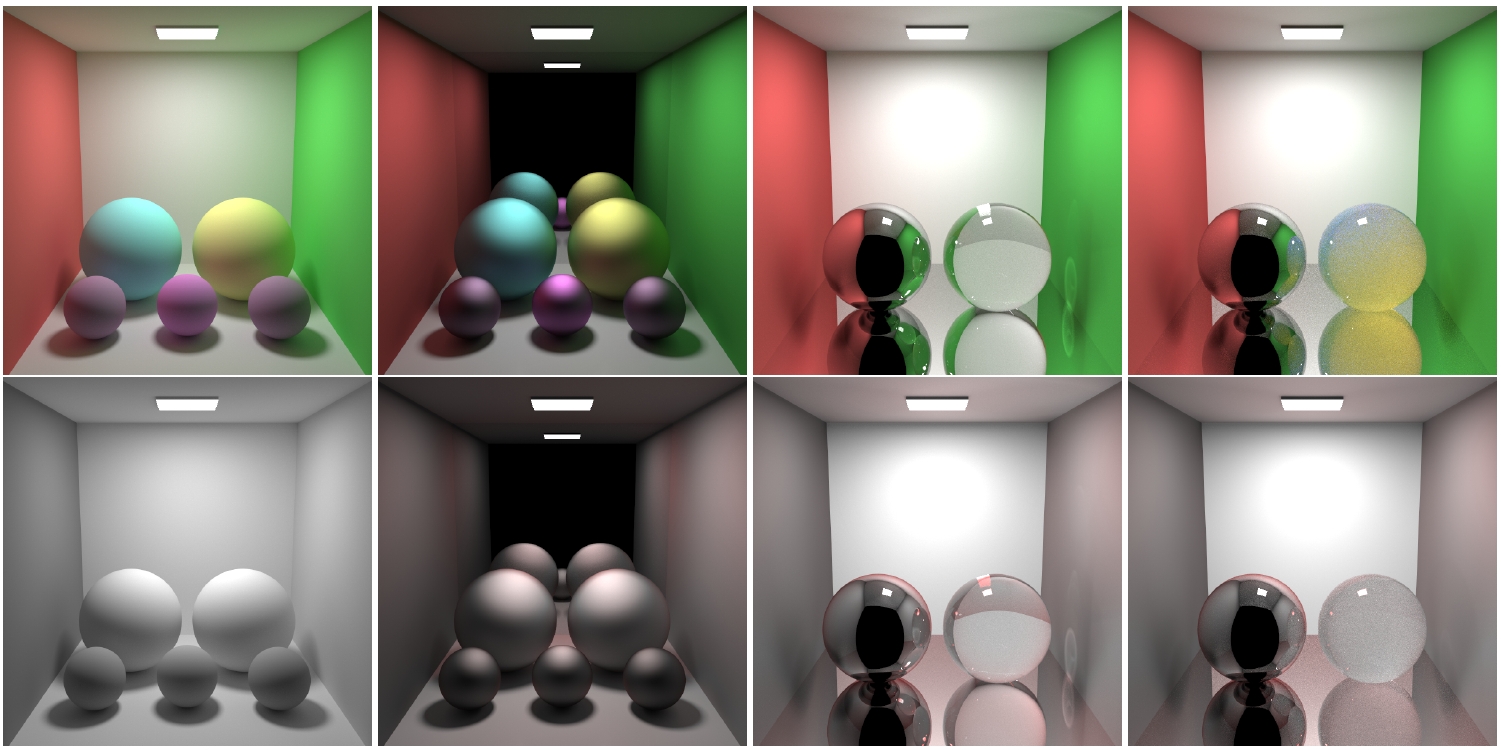
The top row shows the intensity of computed light, bottom row shows the scene in greyscale with degree of polarisation overlaid in red. Scenes from left to right: diffuse, glossy, glass and glass with participating media enabled.

Abstract

While there has been considerable applied research in computer graphics on polarisation rendering, no principled investigation of how the inclusion of polarisation information affects the mathematical formalisms that are used to describe light transport algorithms has been conducted so far. Simple uni-directional rendering techniques do not necessarily require such considerations: but for modern bi-directional light transport simulation algorithms, an in-depth solution is needed.

In this paper, we first define the transport equation for polarised light based on the Stokes Vector formalism. We then define a notion of polarised visual importance, and we show that it can be conveniently represented by a 4x4 matrix, similar to the Mueller matrices used to represent polarised surface reflectance. Based on this representation, we then define the adjoint transport equation for polarised importance. Additionally, we write down the path integral formulation for polarised light, and point out its salient differences from the usual formulation for light intensities. Based on the above formulations, we extend some recently proposed advanced light transport simulation algorithms to support polarised light, both in surface and volumetric transport. In doing that, we point out optimisation strategies that can be used to minimise the overhead incurred by including polarisation support into such algorithms.

Reference

M. Mojzík, T. Skřivan, A. Wilkie, and J. Křivánek. 2016. Bi-directional polarised light transport. In Proceedings of the Eurographics Symposium on Rendering: Experimental Ideas & Implementations (EGSR '16). Eurographics Association, Goslar Germany, Germany, 97-108. DOI: https://doi.org/10.2312/sre.20161215 | BibTeX

Links and Downloads

paper	slides

PDF (7 MB)	PDF (9 MB)

Acknowledgments

This work was financed by the Czech Science Foundation under grant number 16-08111S, as well as by the Grant Agency of Charles University via grant SVV-2016-260332.